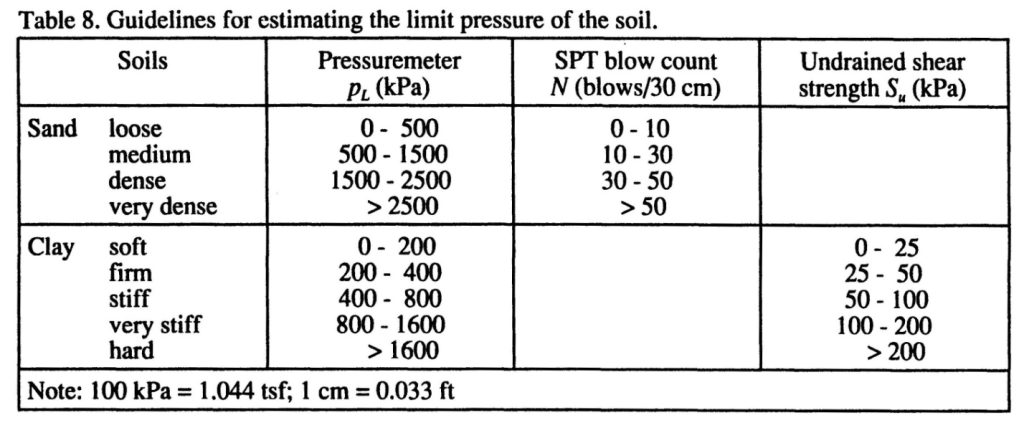
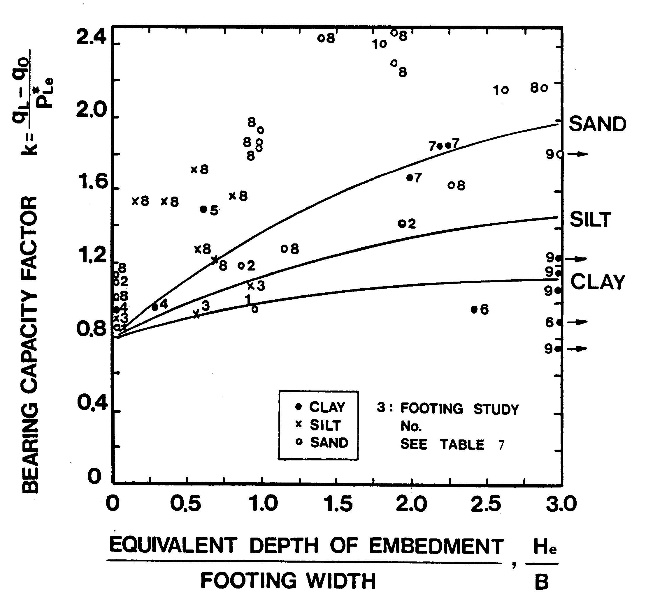
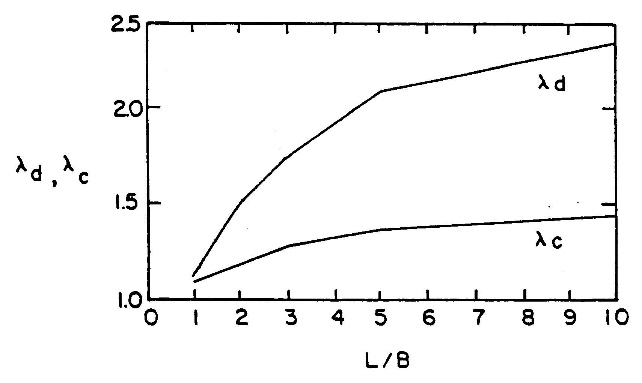
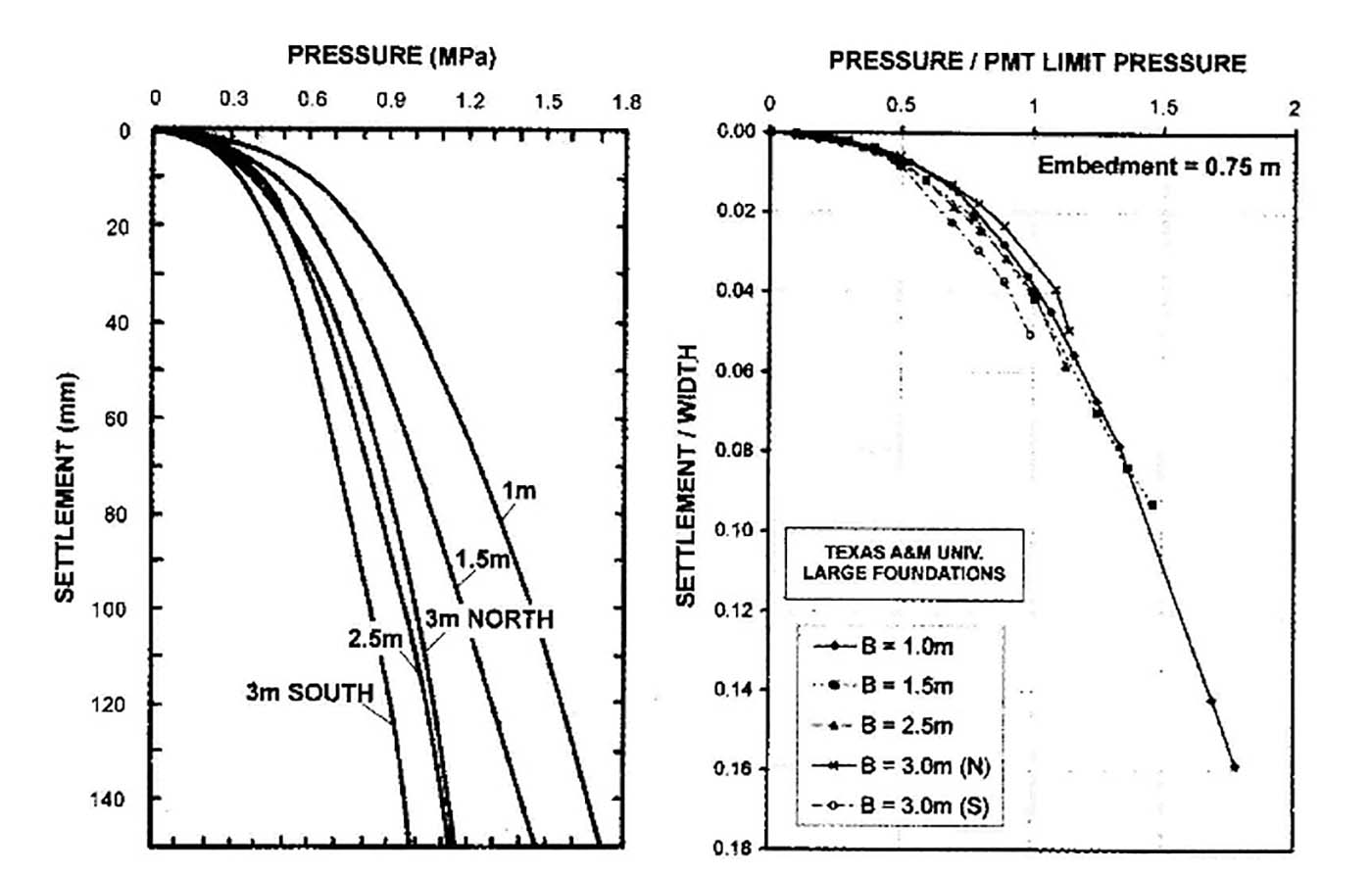
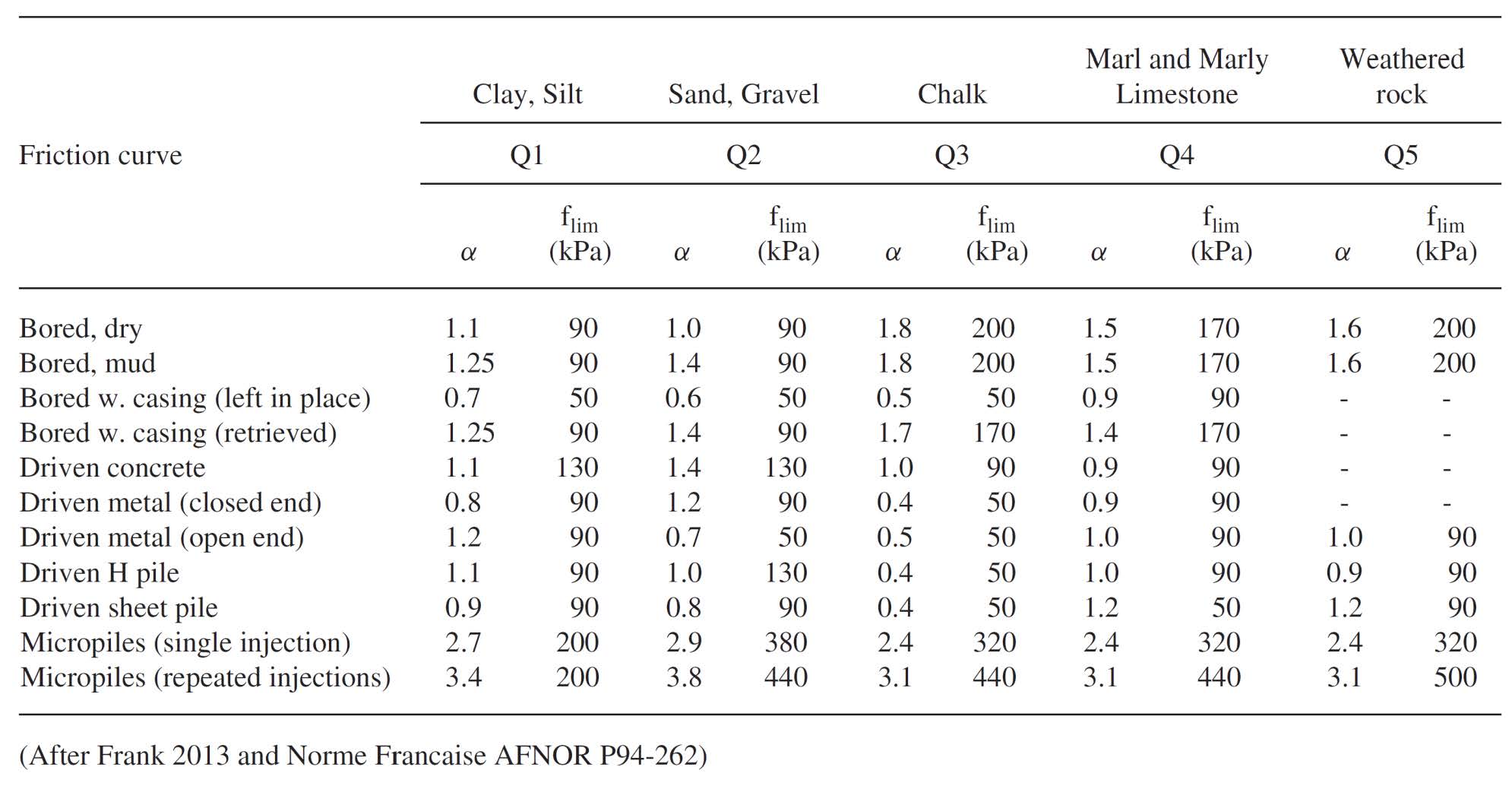
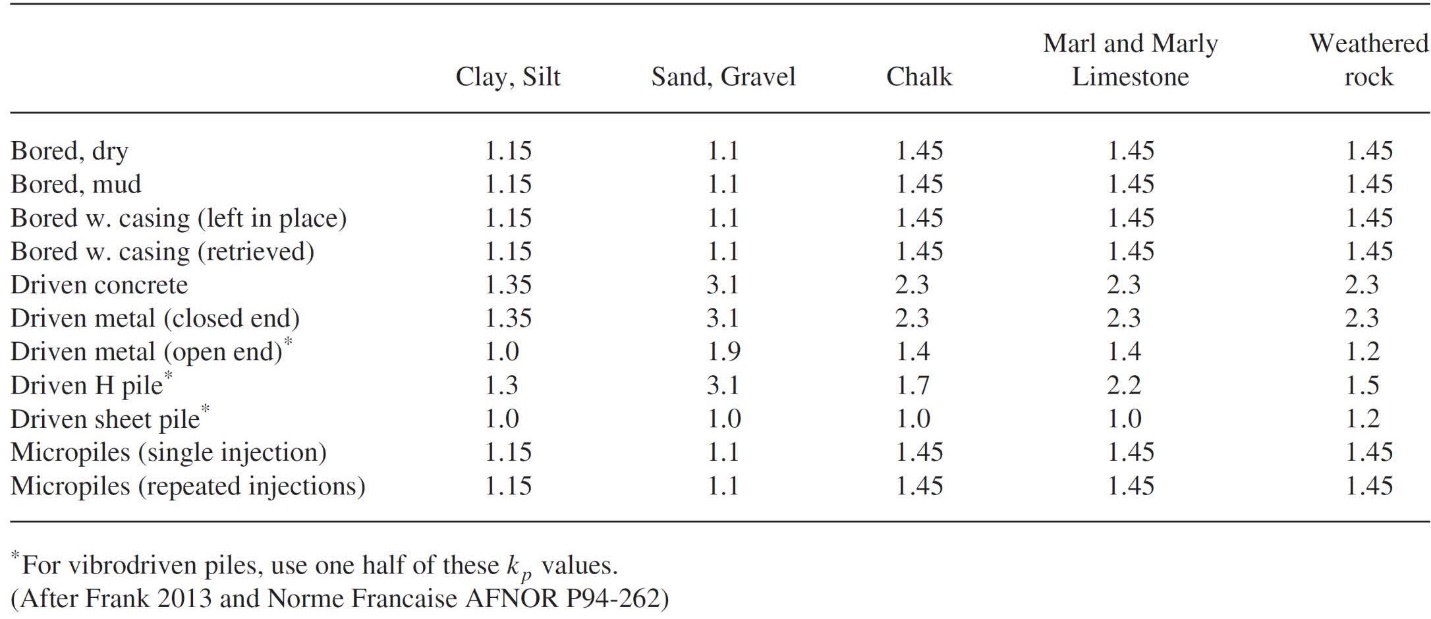
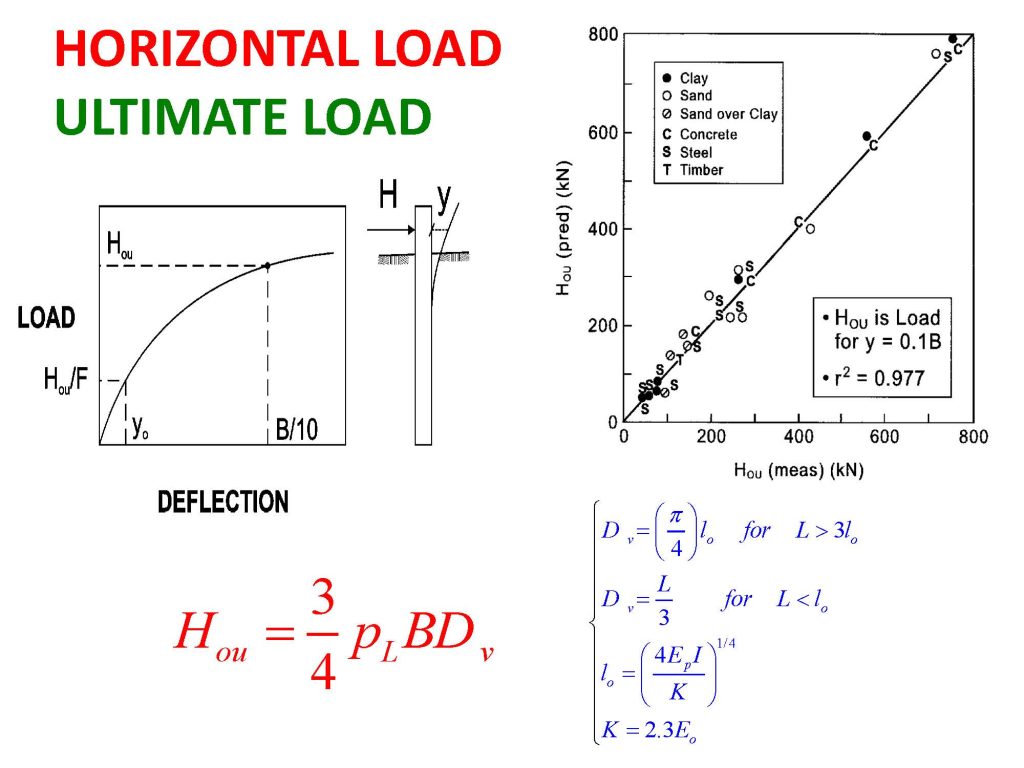
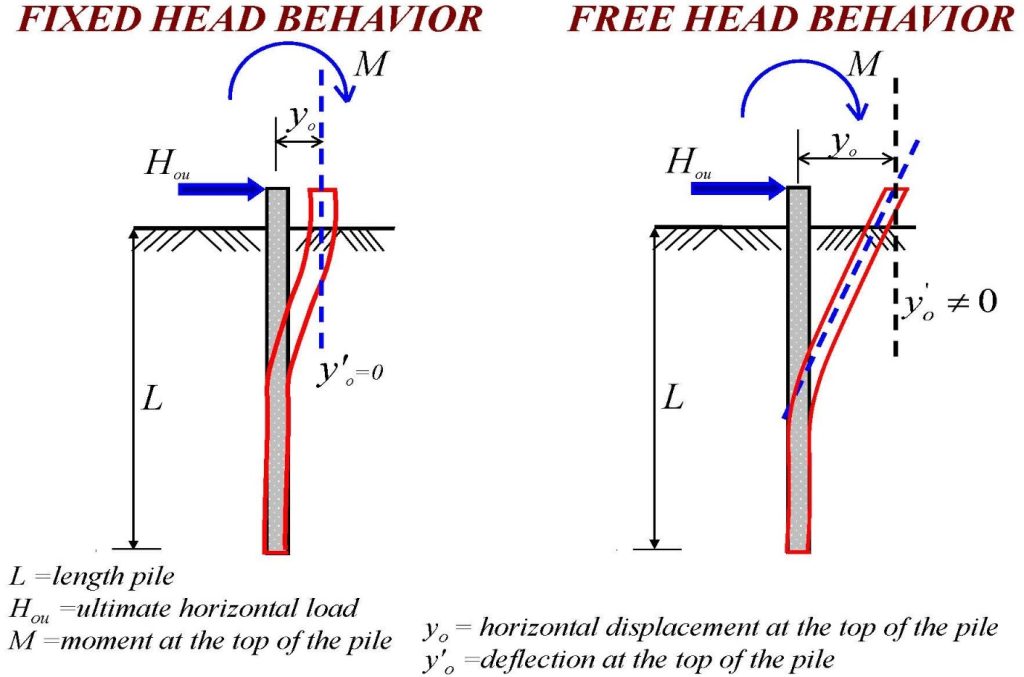
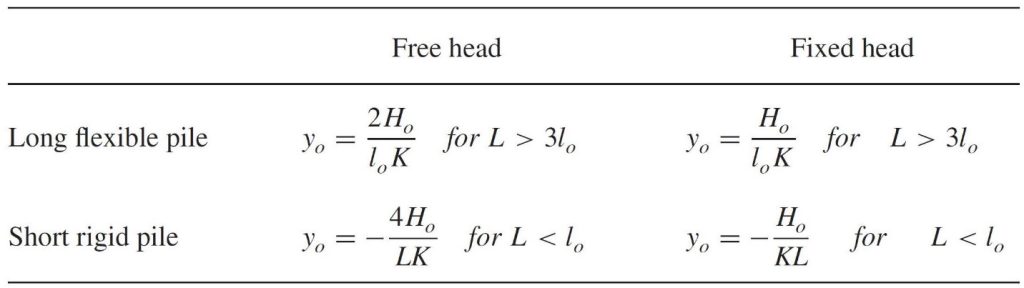
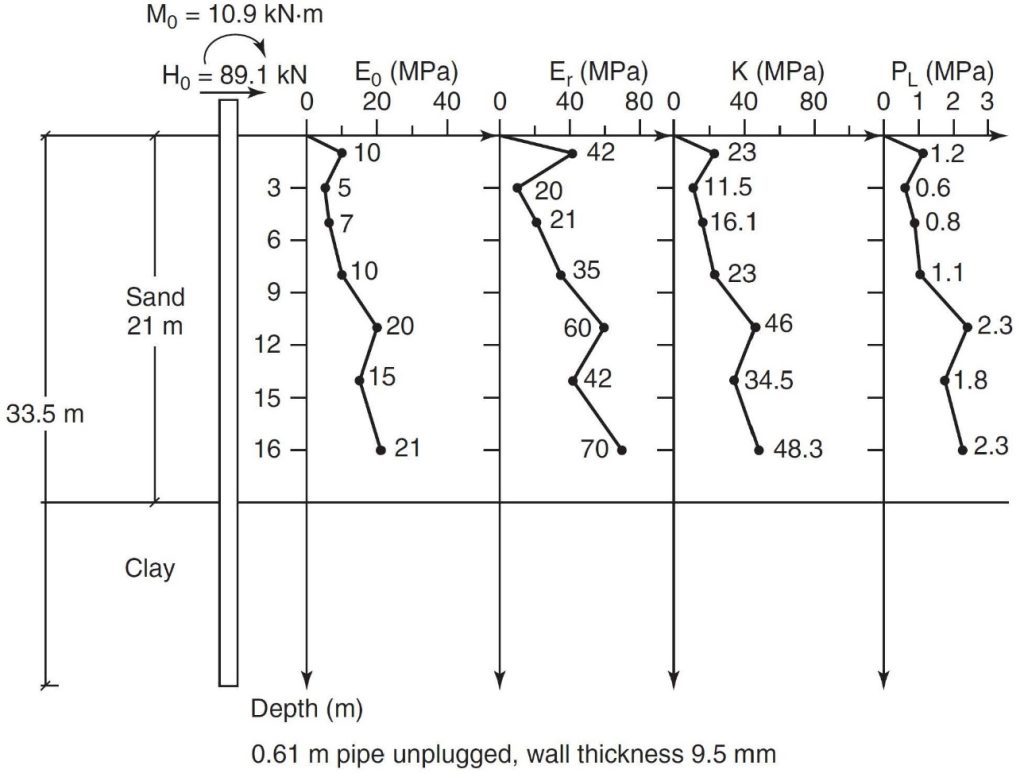
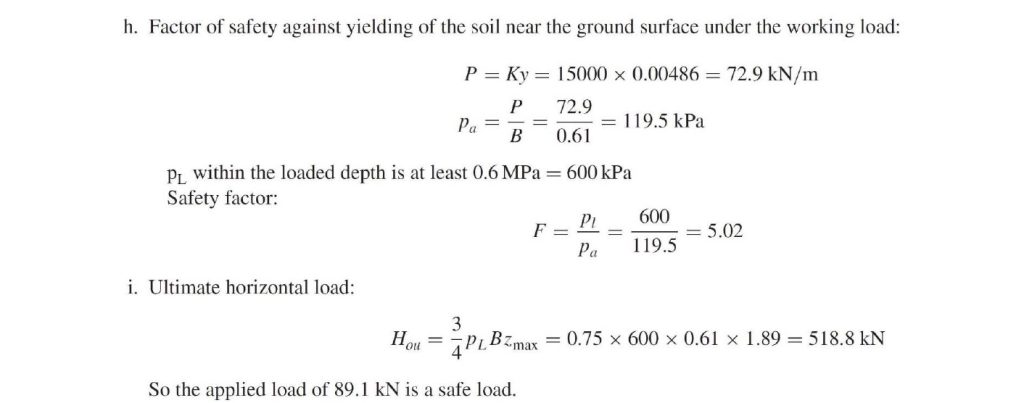
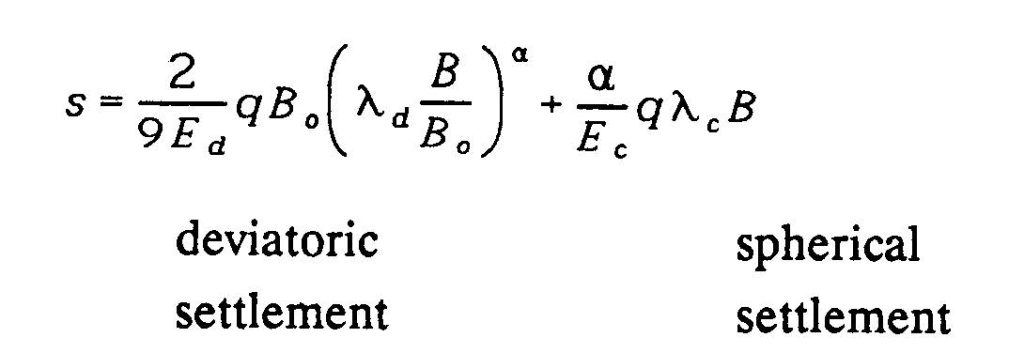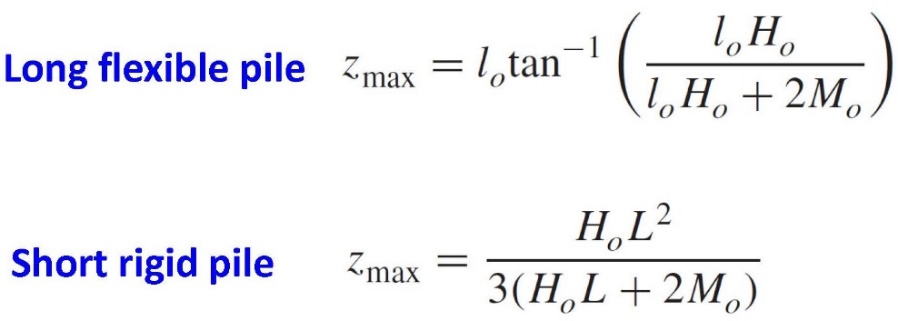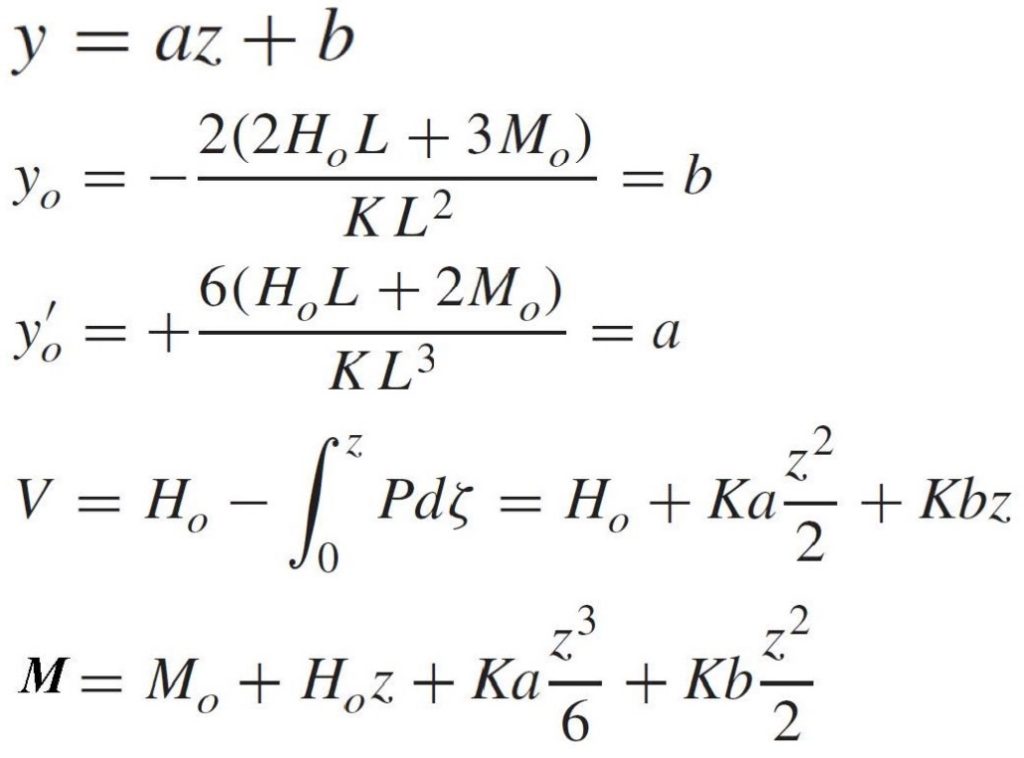Pengujian Pressuremeter (PMT) merupakan metode pengujian tanah in-situ yang digunakan untuk menentukan karakteristik mekanik tanah seperti modulus deformasi (E), koefisien Poisson (ν), dan tekanan pra-konsolidasi (σ'c). Pengujian ini dilakukan dengan memasukkan sebuah probe ke dalam tanah dan kemudian mengembangkannya dengan tekanan udara atau cairan. Dengan mengamati perubahan volume dan tekanan, maka parameter-parameter tanah dapat dihitung.
Elastometer D-4719 adalah salah satu jenis alat ukur yang dapat digunakan dalam pengujian PMT. Alat ini berfungsi untuk mengukur deformasi tanah akibat tekanan yang diberikan oleh probe. Data deformasi yang diperoleh kemudian digunakan untuk menghitung modulus deformasi tanah.
Tujuan Pengujian PMT pada Pondasi Bendungan
- Menentukan Karakteristik Mekanik Tanah: Data PMT sangat penting untuk menentukan karakteristik mekanik tanah pondasi bendungan, yang akan digunakan dalam analisis stabilitas dan perhitungan penurunan tanah.
- Evaluasi Kualitas Grouting: PMT dapat digunakan untuk mengevaluasi efektivitas grouting pada zona kontak antara pondasi dan tubuh bendungan. Jika grouting berhasil, maka modulus deformasi tanah akan meningkat.
- Membandingkan dengan Hasil Laboratorium: Hasil PMT dapat dibandingkan dengan hasil uji laboratorium untuk memverifikasi keakuratan parameter tanah yang digunakan dalam analisis.
- Memantau Perubahan Karakteristik Tanah: PMT dapat dilakukan secara berkala untuk memantau perubahan karakteristik tanah akibat pengaruh beban atau perubahan kondisi lingkungan.
Informasi yang Diperoleh dari Laporan Survey PMT
Laporan survey PMT biasanya berisi informasi berikut:
- Data Umum: Informasi proyek, lokasi pengujian, tanggal pengujian, dan nama personel yang terlibat.
- Deskripsi Lapangan: Deskripsi kondisi lapangan saat pengujian, termasuk jenis tanah, kedalaman air tanah, dan aksesibilitas lokasi pengujian.
- Prosedur Pengujian: Detail prosedur pengujian, termasuk jenis probe yang digunakan, tekanan pengujian, dan frekuensi pengukuran data.
- Hasil Pengujian: Data mentah hasil pengujian, termasuk kurva tekanan-deformasi, dan hasil perhitungan parameter tanah (E, ν, σ'c).
- Analisis Data: Analisis data hasil pengujian, termasuk evaluasi kualitas data, perbandingan dengan hasil laboratorium, dan interpretasi terhadap kondisi tanah.
- Kesimpulan dan Rekomendasi: Kesimpulan mengenai karakteristik mekanik tanah berdasarkan hasil pengujian, serta rekomendasi untuk desain dan analisis struktur.
Pentingnya Data PMT dalam Analisis Stabilitas Bendungan
Data PMT sangat penting dalam analisis stabilitas bendungan karena:
- Perhitungan Tegangan dan Deformasi: Data PMT digunakan untuk menghitung tegangan dan deformasi dalam tanah pondasi akibat beban bendungan.
- Evaluasi Kestabilan: Hasil perhitungan tegangan dan deformasi digunakan untuk mengevaluasi faktor keamanan terhadap berbagai jenis kegagalan, seperti longsor dan penurunan.
- Perancangan Sistem Drainase: Data PMT dapat digunakan untuk merancang sistem drainase yang efektif untuk mengurangi tekanan pori dalam tanah dan meningkatkan stabilitas bendungan.
Langkah-Langkah Pengujian PMT
Pengujian Pressuremeter (PMT) merupakan metode yang cukup kompleks, namun secara garis besar langkah-langkahnya adalah sebagai berikut:
-
Persiapan Lapangan:
- Pemilihan Lokasi: Tentukan lokasi pengujian yang representatif terhadap kondisi tanah yang akan diuji.
- Persiapan Lubang Bor: Bor lubang dengan diameter yang sesuai dengan probe PMT.
- Pemasangan Probe: Masukkan probe PMT ke dalam lubang bor hingga mencapai kedalaman yang diinginkan.
-
Pengujian:
- Inflasi Probe: Pompa udara atau cairan ke dalam probe secara bertahap untuk meningkatkan tekanan.
- Pengukuran Deformasi: Ukur deformasi tanah akibat tekanan yang diberikan. Deformasi ini biasanya diukur menggunakan sensor yang terpasang pada probe.
- Pengambilan Data: Rekam data tekanan dan deformasi secara kontinu selama proses pengujian.
-
Analisis Data:
- Pembuatan Kurva Tekanan-Deformasi: Plotkan data tekanan dan deformasi untuk mendapatkan kurva karakteristik tanah.
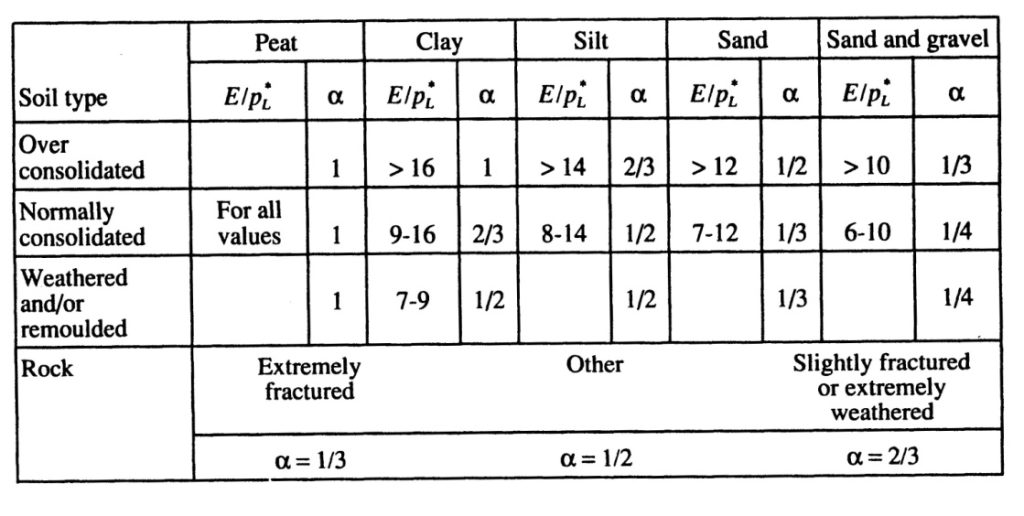
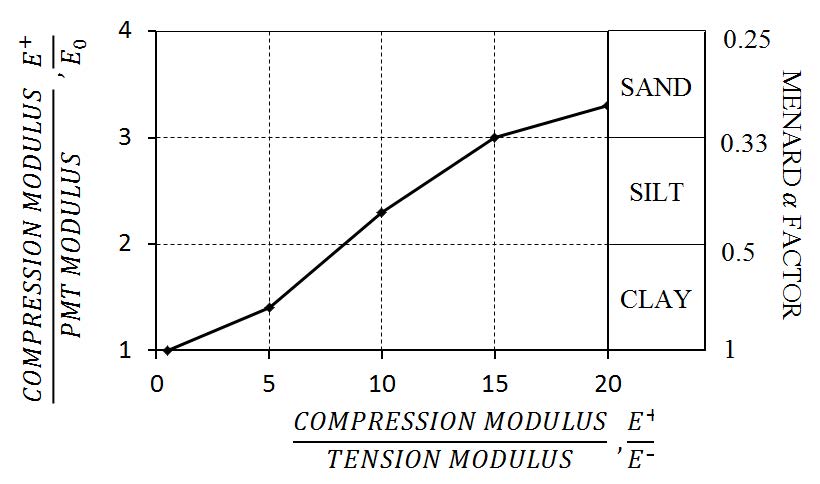
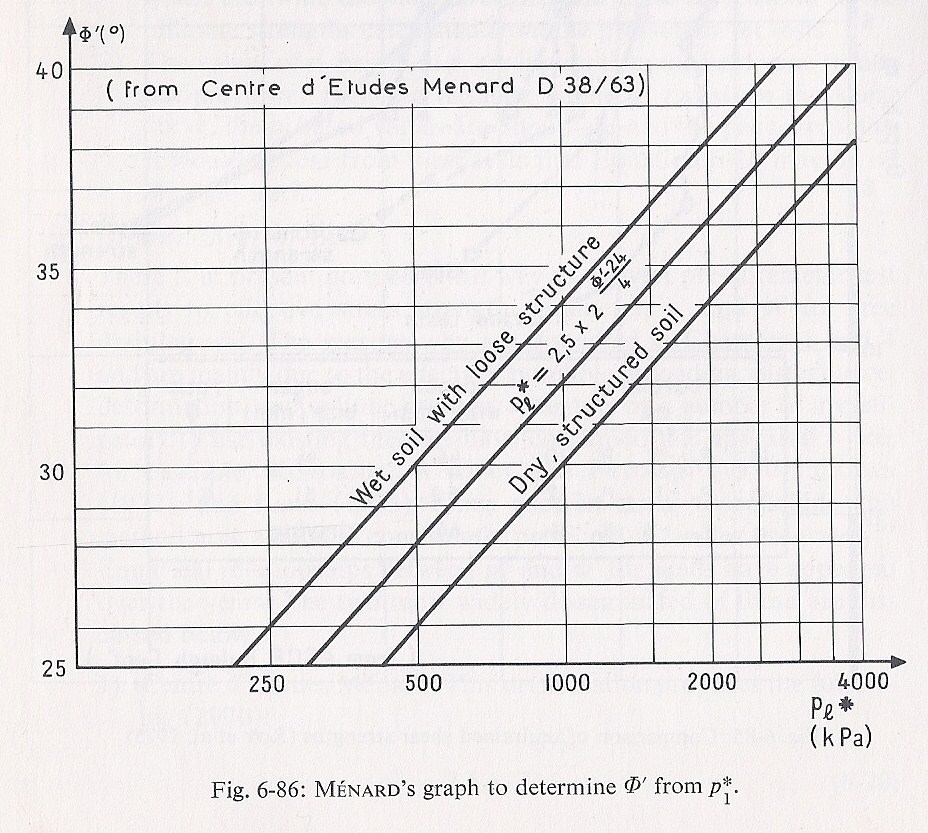
- Penentuan Parameter Tanah: Hitung parameter tanah seperti modulus deformasi (E), koefisien Poisson (ν), dan tekanan pra-konsolidasi (σ'c) berdasarkan kurva tekanan-deformasi.
-
Interpretasi Hasil:
- Evaluasi Kualitas Data: Periksa kualitas data untuk memastikan tidak ada kesalahan atau anomali.
- Bandingkan dengan Data Laboratorium: Bandingkan hasil PMT dengan hasil uji laboratorium untuk memverifikasi keakuratan data.
- Interpretasi Kondisi Tanah: Analisis parameter tanah yang diperoleh untuk mendapatkan gambaran mengenai kondisi tanah di lapangan.
Jenis-Jenis Probe PMT
Terdapat beberapa jenis probe PMT yang umum digunakan, antara lain:
- Probe Selinder: Probe berbentuk silinder yang paling umum digunakan. Probe ini menghasilkan data yang relatif akurat untuk tanah dengan kondisi normal.
- Probe Menerus: Probe yang memiliki panjang yang lebih panjang dibandingkan dengan diameternya. Probe ini cocok untuk mengukur profil modulus deformasi tanah secara vertikal.
- Probe Khusus: Probe yang dirancang khusus untuk kondisi tanah tertentu, misalnya tanah berpasir atau tanah lempung yang sangat lunak.
Faktor-Faktor yang Mempengaruhi Hasil Pengujian PMT
- Jenis Tanah: Jenis tanah sangat mempengaruhi perilaku tanah terhadap tekanan. Tanah berpasir akan memberikan respons yang berbeda dengan tanah lempung.
- Kadar Air: Kadar air dalam tanah akan mempengaruhi kekuatan dan kekakuan tanah.
- Struktur Tanah: Struktur tanah seperti lapisan tanah, retakan, dan akar tanaman dapat mempengaruhi hasil pengujian.
- Kedalaman Pengujian: Kedalaman pengujian akan mempengaruhi nilai parameter tanah yang diperoleh.
- Kecepatan Inflasi: Kecepatan inflasi probe dapat mempengaruhi hasil pengujian, terutama untuk tanah yang sensitif terhadap kecepatan pembebanan.
- Kalibrasi Alat: Keakuratan alat ukur sangat penting untuk mendapatkan hasil pengujian yang akurat.
Analisis Numerik Menggunakan Data PMT
Data PMT dapat digunakan sebagai input dalam analisis numerik, seperti analisis elemen hingga (finite element analysis). Dengan mengintegrasikan data PMT ke dalam model numerik, kita dapat:
- Memprediksi Perilaku Tanah: Memprediksi deformasi dan tegangan dalam tanah akibat beban yang bekerja.
- Mengevaluasi Stabilitas Struktur: Mengevaluasi stabilitas struktur seperti bendungan, tanggul, dan fondasi.
- Mendesain Sistem Drainase: Mendesain sistem drainase yang efektif untuk mengurangi tekanan pori dalam tanah.
Penerapan PMT dalam Berbagai Jenis Struktur
Pengujian PMT memiliki banyak aplikasi dalam berbagai bidang teknik sipil, antara lain:
- Bendungan: Untuk mengevaluasi kondisi tanah pondasi dan memantau perubahan karakteristik tanah selama masa operasi.
- Jalan Raya: Untuk merancang perkerasan jalan dan mengevaluasi stabilitas lereng.
- Bangunan Tinggi: Untuk mengevaluasi kapasitas dukung tanah dan memantau penurunan tanah akibat beban bangunan.
- Terowongan: Untuk mengevaluasi kondisi tanah di sekitar terowongan dan memantau deformasi terowongan.
- Sampah: Untuk mengevaluasi karakteristik mekanik tanah penimbunan sampah.
Saran untuk melaksanakan pengujian PMT agar mendapatkan data yang akurat:
Persiapan Sebelum Pengujian
- Pilih Lokasi yang Tepat: Lokasi pengujian harus representatif terhadap kondisi tanah yang ingin diuji. Hindari area yang terganggu oleh aktivitas konstruksi atau getaran.
- Persiapan Peralatan: Pastikan semua peralatan pengujian dalam kondisi baik dan terkalibrasi. Ini termasuk probe PMT, alat pengukur tekanan, dan alat perekam data.
- Pelajari Prosedur: Pahami secara detail prosedur pengujian yang akan dilakukan. Ini akan membantu menghindari kesalahan saat pelaksanaan.
- Dokumentasi: Persiapkan formulir data pengujian untuk mencatat semua data yang diperoleh selama pengujian.
Pelaksanaan Pengujian
- Kedalaman Pengujian: Tentukan kedalaman pengujian yang sesuai dengan tujuan pengujian. Biasanya, beberapa kedalaman dilakukan untuk mendapatkan profil karakteristik tanah.
- Kecepatan Inflasi: Atur kecepatan inflasi probe sesuai dengan jenis tanah dan tujuan pengujian. Kecepatan yang terlalu cepat dapat menyebabkan hasil yang tidak akurat, terutama untuk tanah yang sensitif terhadap kecepatan pembebanan.
- Pengukuran Deformasi: Pastikan sensor deformasi terpasang dengan benar dan tidak terganggu selama pengujian.
- Pengambilan Data: Rekam data tekanan dan deformasi secara kontinu dan akurat.
- Ulangi Pengujian: Lakukan pengujian ulang pada beberapa titik untuk memastikan hasil yang diperoleh konsisten.
Analisis Data
- Pemilihan Metode Analisis: Pilih metode analisis yang sesuai dengan jenis data yang diperoleh dan tujuan pengujian.
- Evaluasi Kualitas Data: Periksa kualitas data untuk memastikan tidak ada data yang anomali atau error.
- Bandingkan dengan Data Laboratorium: Bandingkan hasil PMT dengan hasil uji laboratorium untuk memverifikasi keakuratan data.
- Interpretasi Hasil: Interpretasikan hasil analisis dengan hati-hati. Pertimbangkan faktor-faktor yang dapat mempengaruhi hasil pengujian, seperti jenis tanah, kadar air, dan struktur tanah.
Faktor Tambahan yang Perlu Diperhatikan
- Pengaruh Lingkungan: Faktor lingkungan seperti suhu dan kelembaban dapat mempengaruhi hasil pengujian.
- Pengaruh Waktu: Waktu pelaksanaan pengujian dapat mempengaruhi hasil, terutama untuk tanah yang bersifat time-dependent.
- Keterampilan Operator: Keterampilan operator dalam melakukan pengujian sangat penting untuk mendapatkan hasil yang akurat.
Saran Tambahan
- Konsultasi dengan Ahli: Konsultasikan dengan ahli geoteknik untuk mendapatkan saran yang lebih spesifik mengenai pelaksanaan pengujian PMT.
- Dokumentasi yang Lengkap: Dokumentasikan semua aspek pengujian, mulai dari persiapan hingga analisis data. Dokumentasi yang lengkap akan sangat berguna untuk evaluasi di kemudian hari.
- Kalibrasi Berkala: Lakukan kalibrasi alat secara berkala untuk memastikan keakuratan pengukuran.
Dengan mengikuti saran-saran di atas, diharapkan Anda dapat memperoleh data PMT yang berkualitas dan akurat, yang selanjutnya dapat digunakan untuk analisis dan perancangan struktur yang lebih baik.
Kesimpulan
Pengujian PMT merupakan alat yang sangat berguna dalam bidang geoteknik. Dengan memahami prinsip kerja dan penerapannya, kita dapat memperoleh informasi yang akurat mengenai kondisi tanah dan menggunakannya untuk perencanaan dan desain struktur yang aman dan ekonomis.
Pengujian PMT merupakan alat yang sangat berguna dalam evaluasi kondisi tanah pondasi bendungan. Data yang diperoleh dari pengujian ini sangat penting untuk memastikan keamanan dan keberlanjutan operasi bendungan.
Penutup
Sekian Penjelasan Singkat Mengenai Pengujian Pressuremeter (PMT) dan Elastometer D-4719 pada Pondasi Bendungan. Semoga Bisa Menambah Pengetahuan Kita Semua.


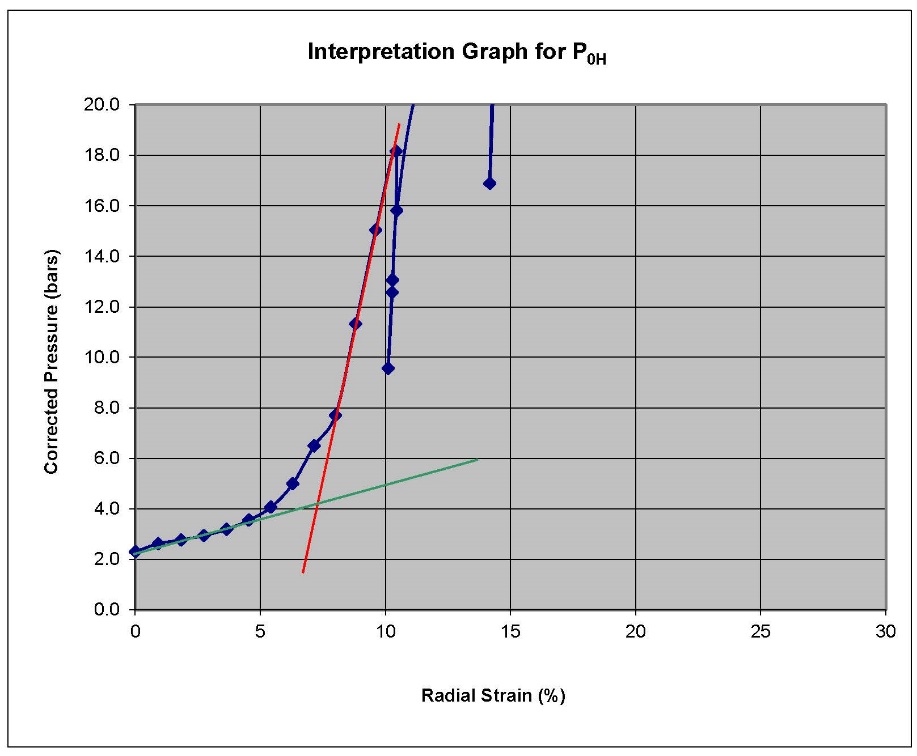
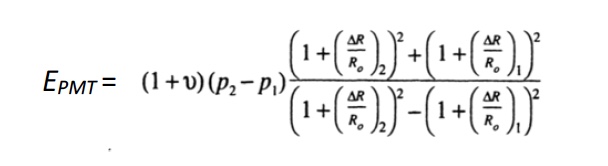
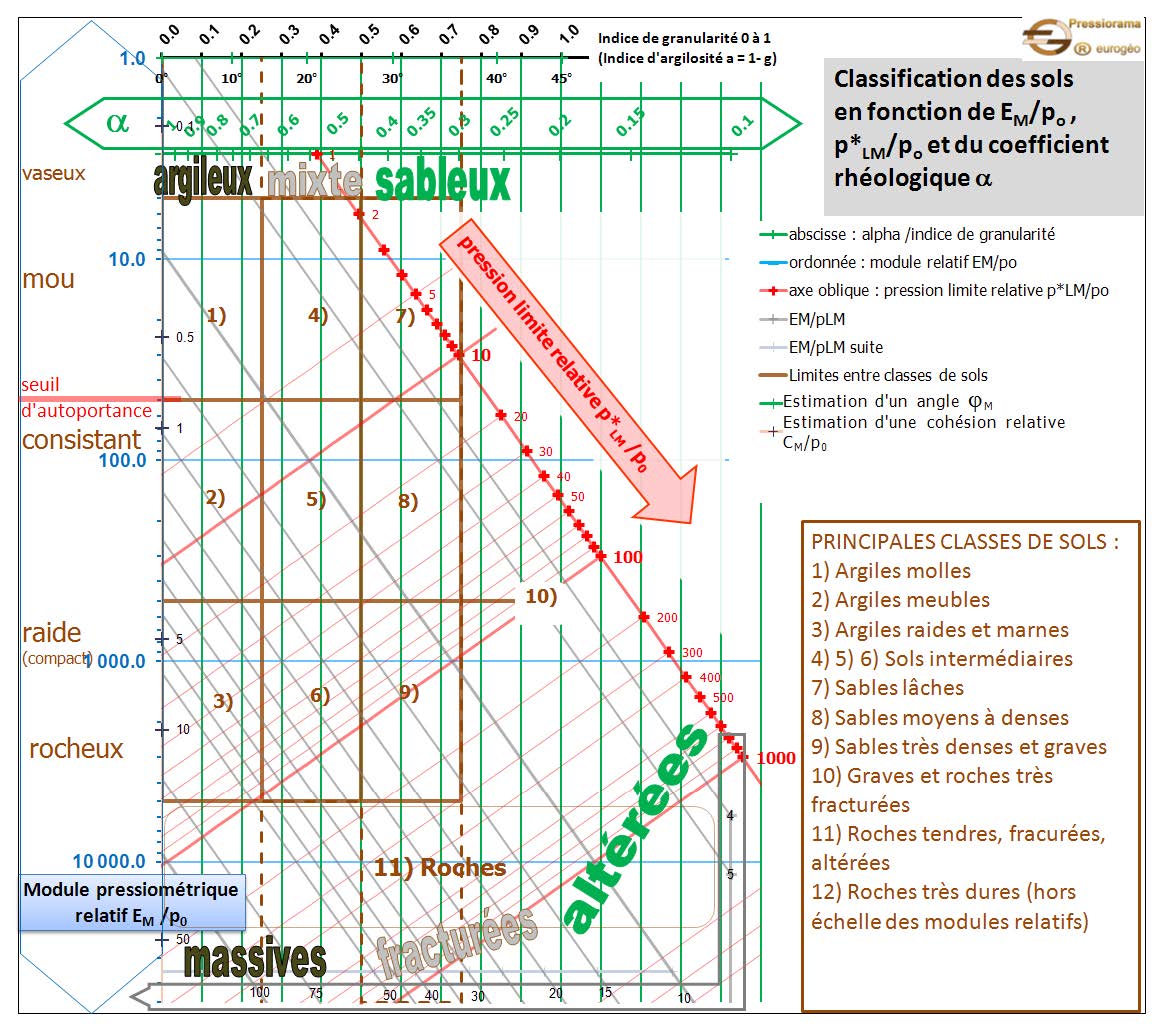
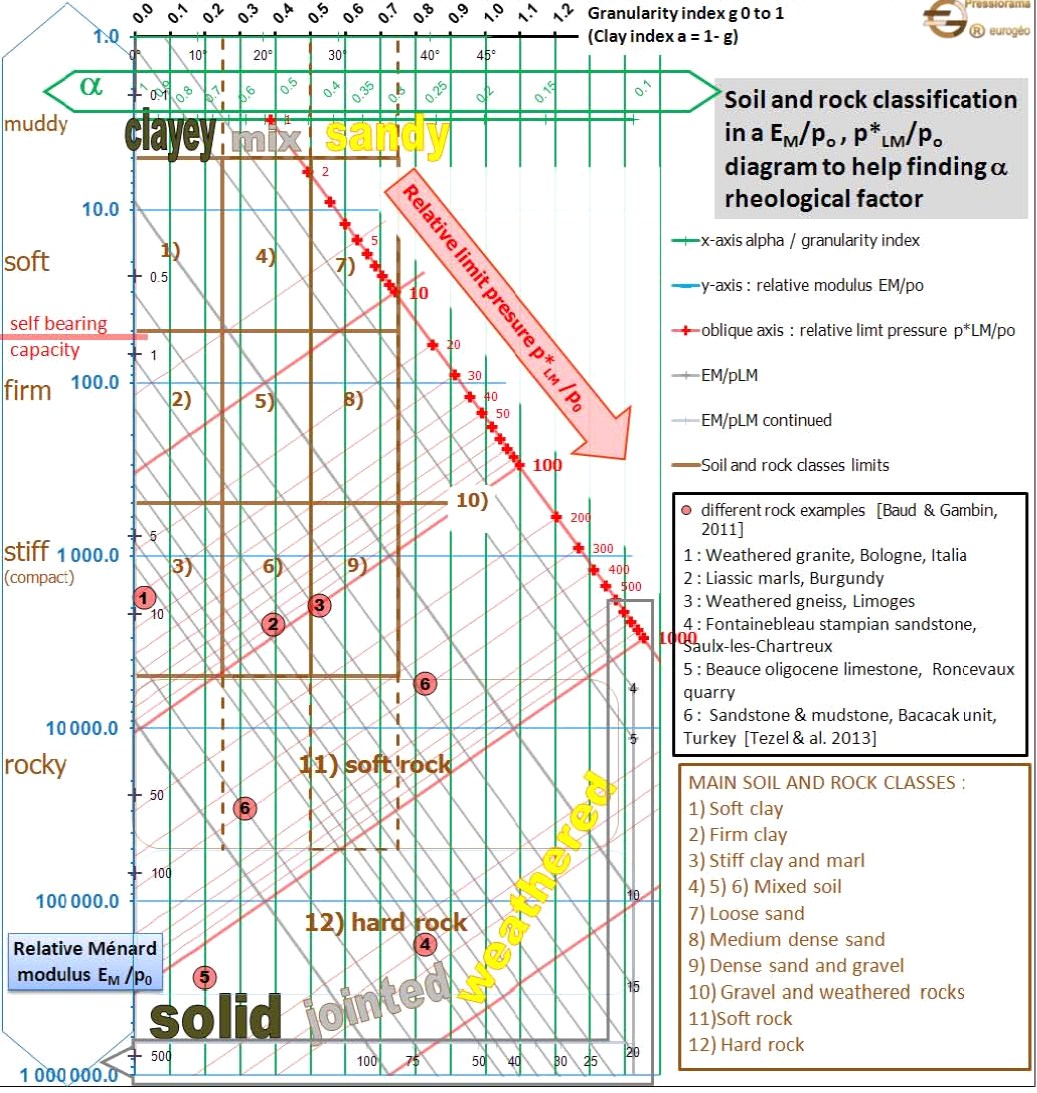

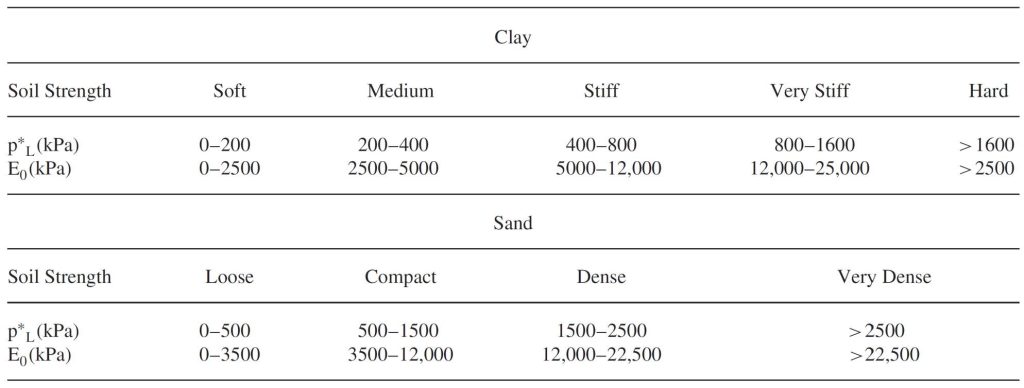
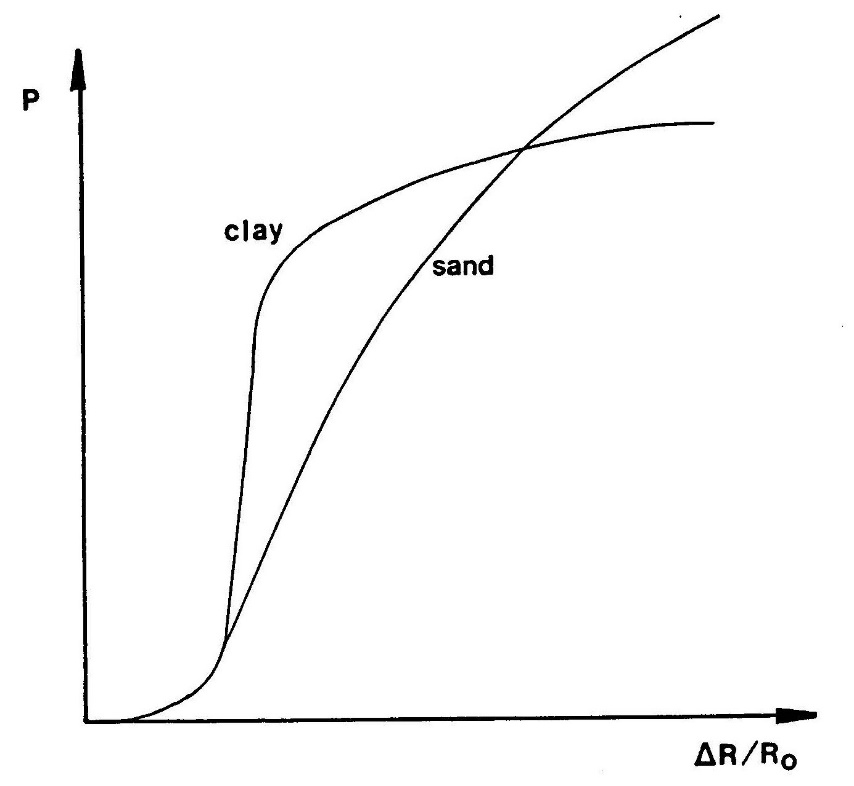
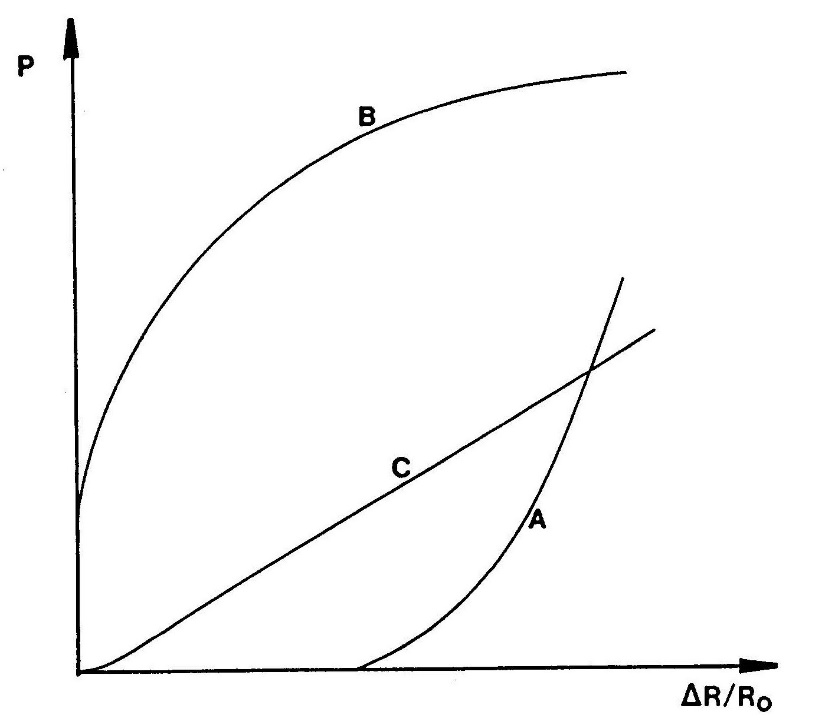
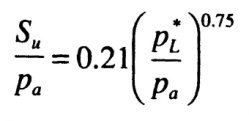
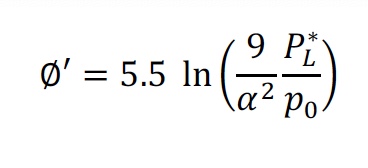
![Modified Pressiorama Chart to compute angle of internal friction [Français]](https://insitusoil.com/wp-content/uploads/2022/02/Soil-pressuremeter-test-figure-17a-modified-pressiorama.png)
![Modified Pressiorama Chart to compute angle of internal friction [English]](https://insitusoil.com/wp-content/uploads/2022/02/Soil-pressuremeter-test-figure-17b-modified-pressiorama.jpg)